STATISTICS: Hypothesis Testing
- 1. HYPOTHESIS TESTING Prepared by Roderico Y. Dumaug, Jr. For Intro to Statistics
- 2. Objectives 1) Able to formulate statistical hypothesis 2) Discuss the two types of errors in hypothesis testing 3) Establish a decision rule for accepting or rejecting a statistical hypothesis at a specified level of significance 4) Distinguish between the one-sample case and two-sample case tests of hypothesis concerning means 5) Choose the appropriate test statistics for a particular set of data.
- 3. Symbols Applicable 1) Ho – Null Hypothesis 2) H1 – Alternative Hypothesis 3) β – Greek Letter Beta which is the probability of committing a Type 2 Error 4) α – Greek letter Alpha which denotes a probability of committing a Type 1 Error and is known as the Level of Significance 5) z 6) σ – Greek letter Sigma which means the Variance 7) σx - the standard deviation of the sampling distribution of the mean 8) µ - Greek letter ‘mu’ which is the mean of the normal population 9) n – Sample size 10) - Sample mean 11) t – t distribution; a case where the population standard deviation is unknown 12) s – standard deviation
- 4. Hypothesis Testing: Introduction • Theory of Statistical Inference: Consists of methods which one makes inferences or generalizations about a population. Example is the Tests of Hypothesis. • Population vs. Random Sample
- 5. Statistical Hypothesis • Definition: A statistical hypothesis is an assertion or conjecture concerning one or more populations. An assumption or statement, which may or may not be true concerning one or more population. • Two types of Statistical Hypothesis: a) The NULL HYPOTHESIS, Ho b) The ALTERNATIVE HYPOTHESIS, H1 a) Nondirectional Hypothesis– Asserts that one value is different from another (or others). Also called as the 2-sided Hypothesis. “Not equal to” or ≠. a) Directional Hypothesis – An assertion that one measure is Less than (or greater than) another measure of similar nature. Also called the 1-sided Hypothesis. “<“ or “>”
- 6. Examples of Statistical Hypothesis 1) Ho: The average annual income of all the families in the City is Php36,000 (µ = Php 36,000). H1 : The average annual income of all the families in the City is not Php36,000 (µ ≠ Php36,000). 2) Ho: There is no significant difference between the average life of brand A light bulbs and that of brand B light bulbs (µA = µB). H1 : There is a significant difference between the average life of brand A light bulbs and that of brand B light bulbs (µA ≠ µB). 3) Ho: The proportion of Metro Manila college students who prefer the taste of Papsi Cola is ²/₃(p = ²/₃) H1 : The proportion of Metro Manila college students who prefer the taste of Papsi Cola is less than ²/₃(p < ²/₃). 4) Ho: The proportion of TV viewers who watch talk shows from 9:00 to 10:00 in the evening is the same on Wednesday and Fridays (p1 = p2) H1 : The proportion of TV viewers who watch talk shows from 9:00 to 10:00 in the evening is greater on Wednesday than on Fridays (p1 > p2).
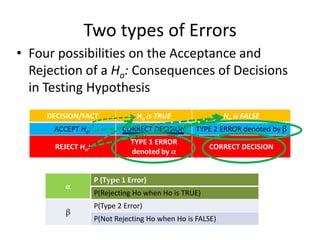
- 7. Two types of Errors • Four possibilities on the Acceptance and Rejection of a Ho: Consequences of Decisions in Testing Hypothesis DECISION/FACT Ho is TRUE Ho is FALSE ACCEPT Ho: CORRECT DECISION TYPE 2 ERROR denoted by β TYPE 1 ERROR REJECT Ho: CORRECT DECISION denoted by α P (Type 1 Error) α P(Rejecting Ho when Ho is TRUE) P(Type 2 Error) β P(Not Rejecting Ho when Ho is FALSE)
- 8. Elements of a Test of a Hypothesis • Null Hypothesis (Ho) • Alternative Hypothesis (H1) • Test Statistic: A sample statistic used to decide whether to reject the null hypothesis • Rejection Region • Calculation of Test Statistic • Conclusion: Numerical Value falls in the Rejection Region or not
- 9. Level of Significance • To specify the Probability of committing a Type 1 Error, α, which is popularly known as the Level of Significance • We can determine the Critical Values which define the: – Region of Rejection (or Critical Region) and – Region of Acceptance • The Critical Value serves as the basis for either Accepting or Rejecting a Hypothesis. • When α = .05, the Region of Rejection is 0.05 and the Region of Acceptance is 0.95
- 10. One-Tailed and Two Tailed Tests • Where H1 is Directional, One-Tailed Test • Where H1 is Non-Directional, Two-Tailed Test TYPE OF TESTS DIFFERENCE Region of Rejection lies entirely in one end of the distribution. One-Tailed Test Hypothesizing a Range of Values Involves a Critical Region which is split into two equal parts Two Tailed Test placed in each tail of the distribution. A value of the parameter is being hypothesized. Mathematical Formulation of H1 Region of Rejection Area of Rejection is placed entirely in Greater Than ( >) the Right Tail of the Distribution Less Than ( < ) Region of Rejection is in the Left Tail Both Tails contain Equal areas serving as Not Equal To (≠) Critical Regions
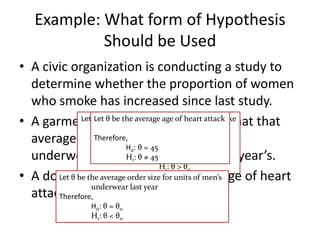
- 11. Example: What form of Hypothesis Should be Used • A civic organization is conducting a study to determine whether the proportion of women who smoke has increased since last study. • A garment θmanufacturer of heart attack that that suspects Let Let θthe the average of women who smoke be be proportion age during the last study average order size for units of men’s Therefore, H : θTherefore, o = 45 underwear has decreased θ H : θ ≠ 45 H : θ = from last year’s. 1 o o 1 oH:θ>θ • A doctor claims thatsize for average age of heart Let θ be the average order the units of men’s underwear last year attack patient is 45. Therefore, Ho: θ = θo H1: θ < θo
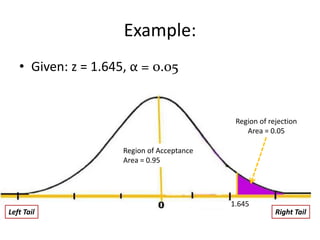
- 12. Example: • Given: z = 1.645, α = 0.05 Region of rejection Area = 0.05 Region of Acceptance Area = 0.95 1.645 Left Tail Right Tail
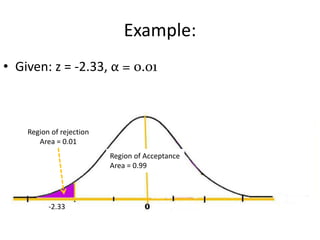
- 13. Example: • Given: z = -2.33, α = 0.01 Region of rejection Area = 0.01 Region of Acceptance Area = 0.99 -2.33
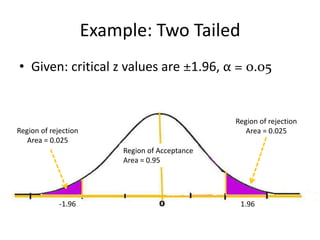
- 14. Example: Two Tailed • Given: critical z values are ±1.96, α = 0.05 Region of rejection Region of rejection Area = 0.025 Area = 0.025 Region of Acceptance Area = 0.95 -1.96 1.96
- 15. Critical Regions In Testing Hypothesis • Rejecting Ho Level of Significance Type of Test One-Tailed Two-Tailed Computed value of z is GREATER z > zo z > zo than the Critical Value Reject Ho Computed value of z is LESS than z < - zo z < -zo the Negative Critical Value
- 16. Steps in Hypothesis Testing 1) Formulate the Ho and the H1 2) Specify the level of significance α 3) Choose the appropriate test statistic 4) Establish the critical region 5) Compute for the value of the statistical test 6) Make a decision and, if possible, draw a conclusion
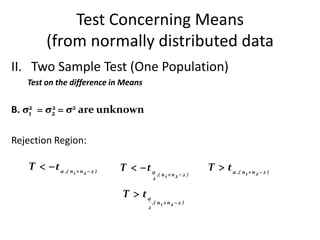
- 17. Test Concerning Means (from normally distributed data) OUTLINE I. One Sample Test (One Population) A. σ2 is known (assume that the population variance is known) B. σ2 is unknown (the population variance is unknown) II. Two Sample Test (One Population) 2 2 A. σ1 and σ2 are known B. σ1 = σ2 = σ2 are unknown 2 2 C. k sample test
- 18. Test Concerning Means (from normally distributed data I. One Sample Test (One Population) A. σ2 is known (assume that the population variance is known) Conditions: We hypothesized that the MEAN of a Normal Population with a variance of σ2 is µo . We take a random sample of size n from this population and obtain a sample mean of which is somewhat different from µo . To determine whether or not the observed difference between the computed value and the hypothesized µo is significant, we formulate the following hypothesis. 1) Ho: µ = µo 2) Ho: µ = µo 3) Ho: µ = µo H1 : µ < µo H1 : µ ≠ µo H1 : µ >µo
- 19. Test Concerning Means (from normally distributed data A. σ2 known (assume that the population variance is known) Since the parameter σ is known, the Z statistics is employed as the test statistics. Consequently, the z score corresponding to is: x o z x where the denominator σx represents the standard error of the mean ( or the standard deviation of the sampling distribution of the mean) and is computed by the formula: Supposed α = 0.05 and the critical values are x 1.96 and -1.96 then the ff decision rules applies: n 1. Reject Ho and accept H1, if z > 1.96 or z < -1.96 2. Cannot reject Ho (Accept H1), if z is within the interval between -1.96 and 1.96
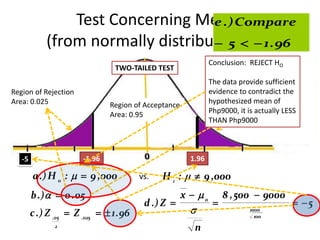
- 20. Test Concerning Means (from normally distributed data A. σ2 known (assume that the population variance is known) Rejection Region: Z Z Z Z Z Z 2 Z Z 2
- 21. Test Concerning Means e .) Compare (from normally distributed 1 . 96 5 data Conclusion: REJECT HO A. σ2 known (assume that the population variance is known) TWO-TAILED TEST The data provide sufficient Example: One community college hypothesized that theRegion of Rejection the Region of Rejection evidence starting monthly mean to contradict Area: 0.025 of its graduatesRegion of Acceptance Area: 0.025 hypothesized mean of salary is Php9000 and a stand deviation of Php1,000. A Php9000, it is actually LESS sample of 100 graduates were questioned and it was found that the average Area: 0.95 THAN Php9000 starting salary is Php8,500.00. Test this hypothesis at 5% level of significance. Given: µo = 9,000 σ = 1,000 n = 100 x 8 , 500 -5 -1.96 1.96 a .) H o : 9 ,000 vs. H 1 : 9 ,000 b .) 0 . 05 x o 8 , 500 9000 d .) Z 5 c .) Z .05 Z .025 1 . 96 1000 100 2 n
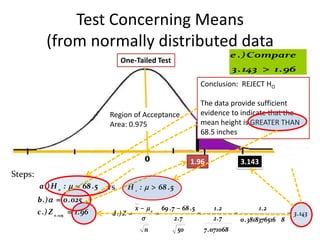
- 22. Test Concerning Means (from normally distributed data e .) Compare One-Tailed Test A. σ2 known (assume that the population variance is known) 3 . 143 1 . 96 Conclusion: REJECT HO Region of Rejection Example: The average height of males in the freshmen class of a certain college Area: 0.025 The data provide sufficient has been 68.5 inches, with a standard deviation of 2.7 inches. Is there a reason to believe thatRegion has been an increase in theto indicateheight if a there of Acceptance evidence average that the random sample of 50 Area: 0.975 present freshmen heighthave an average males in the mean class is GREATER THAN 68.5 inches height 69.7 inches? Test at 0.025 level of significance. Given: µo = 68.5 σ= 2.7 x 69 . 7 1.96 3.143 Steps: a .) H o : 68 . 5 vs. H 1 : 68 . 5 b .) 0 . 025 x o 69 . 7 68 . 5 1.2 1.2 c .) Z 0 .025 1 . 96 d .) Z 3 . 143 2 .7 2 .7 0 . 3818376516 8 n 50 7 . 071068
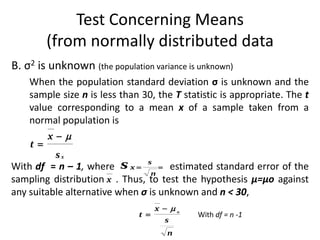
- 23. Test Concerning Means (from normally distributed data B. σ2 is unknown (the population variance is unknown) When the population standard deviation σ is unknown and the sample size n is less than 30, the T statistic is appropriate. The t value corresponding to a mean x of a sample taken from a normal population is x t sx With df = n – 1, where s x s estimated standard error of the n sampling distribution x . Thus, to test the hypothesis µ=µo against any suitable alternative when σ is unknown and n < 30, x o t With df = n -1 s n
- 24. Test Concerning Means (from normally distributed data B. σ2 is unknown (the population variance is unknown) Rejection Region: T t ,( n 1 ) T t T t ,( n 1 ) ,( n 1 ) 2 T t ,( n 1 ) 2
- 25. Test Concerning Means (from normally distributed data One-Tailed Test e .) Compare B. σ2 is unknown (the population variance is unknown) 3 . 06 2 . 821 Example: A major car manufacturer wants to test a new engine to see whether it meets new air Conclusion: REJECT HO pollution standards. The mean µ of all engines of this type must be less than 20 parts Region million of carbon. Ten engines are manufactured for testing purposes, and the per of Rejection Area: 0.01and standard deviation of the emission for this sample of engines were mean The data provide sufficient determined to be: evidence that the engine type Region of Acceptance Area: 0.99 meets pollution control x 17 . 1 parts / million s = 3.0 parts/million Do the data supply evidence to allow the manufacturer to conclude that this type of engine meets the pollution standard? Assume that the manufacturer is willing to risk a Type 1 -3.06 with -2.821 error probability α = 0.01. Given: µo = 20 n = 10 x 17 . 1 s = 3.0 a .) H o : 20 vs . H 1 : 20 b .) 0 . 01 x 0 17 . 1 20 c .) t ,( n 1 ) t 0 .01 ,( 10 1 ) t 0 .01 , 9 2 . 821 d .) T 3 . 06 s 3 .0 n 10
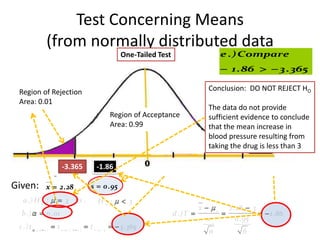
- 26. Test Concerning Means (from normally distributed data One-Tailed Test e .) Compare B. σ2 is unknown (the population variance is unknown)1 . 86 3 . 365 Example: Suppose a pharmaceutical company must demonstrate that a prescribed dose of a certain new drug DO NOT REJECTinO Region of Rejection Conclusion: will result H average increase in blood pressure of lessThe data3do not provide Area: 0.01 than points. Assume that only six patients can Acceptance in the sufficientphase of human Region of be used initial evidence to conclude n x ( x ) Area:(0.99 35 . 79 ) 187 . 69 214 . 74 187 . 69 2 2 6 )( s testing. Result: the six patients have blood pressureincrease in of that mean increase the 1.7, 3.0, 1 ) 3.4, 2.7, and 2.1 points. Use the resulting from n ( n 0.8, 30 30 blood pressure results to determine if there is evidence that the taking the drugsatisfies the 27 . 05 new drug is less than 3 s requirement .that the resulting increase in blood pressure 0 901666 0 . 95 30 -3.365 -1.86 averages less than 3 points. Given: x 2 .28 s 0 . 95 a .) H o : 3 vs . H0 : 3 x 0 2 . 28 3 b .) 0 . 01 d .) T 1 . 86 s 0 . 95 c .) t ,( n 1 ) t 0 .01 ,( 6 1 ) t 0 .01 , 5 3 . 365 n 6
- 27. Test Concerning Means (from normally distributed data) OUTLINE I. One Sample Test (One Population) A. σ2 is known (assume that the population variance is known) B. σ2 is unknown (the population variance is unknown) II. Two Sample Test (One Population) 2 2 A. σ1 and σ2 are known B. σ1 = σ2 = σ2 are unknown 2 2 C. k sample test
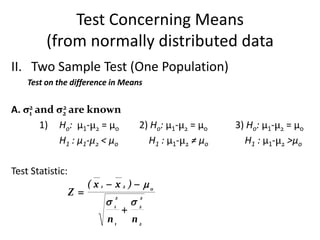
- 28. Test Concerning Means (from normally distributed data II. Two Sample Test (One Population) Test on the difference in Means A. σ2 and σ2 are known 1 2 1) Ho: µ1-µ2 = µo 2) Ho: µ1-µ2 = µo 3) Ho: µ1-µ2 = µo H1 : µ1-µ2 < µo H1 : µ1-µ2 ≠ µo H1 : µ1-µ2 >µo Test Statistic: ( x 1 x 2 ) o Z 1 2 2 2 n1 n2
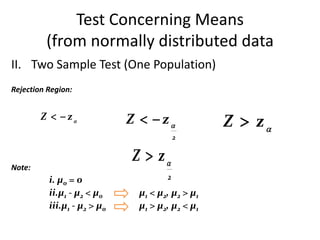
- 29. Test Concerning Means (from normally distributed data II. Two Sample Test (One Population) Rejection Region: Z z Z z Z z 2 Z z Note: i. µo = 0 2 ii.µ1 - µ2 < µ0 µ1 < µ2, µ2 > µ1 iii.µ1 - µ2 > µo µ1 > µ2, µ2 < µ1
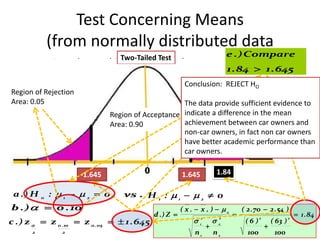
- 30. Test Concerning Means (from normally distributed data Two-Tailed Test e .) Compare II. Two Sample Test (One Population) 1 . 84 1 . 645 Example: A university investigation, conducted to determineConclusion: ownership if students affect whether car REJECT H O their academic achievement, was based on two random samples Regionstudents, each drawn of 100 of Rejection Region of the student body. The average and standard deviation of each group’s GPA (grade point from Rejection Area:average) are as shown. 0.05 Area: 0.05 The data provide sufficient evidence to Region of Acceptance indicate a difference in the mean Non-Car owners (n1=100) Car Owners (n2=100) Area: 0.90 achievement between car owners and GPA GPA non-car owners, in fact non car owners x 1 2 . 70 s 0 . 60 2 . 54 s 0 . performance than x 2 have better academic63 1 2 car owners. Do the data present sufficient evidence to indicate a difference in the mean achievement between car owners and noncar owners? Test using α=0.10 -1.645 1.645 1.84 Define: µ1 = mean GPA for Non-car owners; µ2 = mean GPA for Car owners; µ0 = 0 a .) H 0 : 1 2 0 vs . H 1 : 1 2 0 b .) 0 . 10 ( x 1 x 2 ) 0 ( 2 . 70 2 . 54 ) d .) Z 1 . 84 c .) z z 0 . 10 z 0 . 05 1 . 645 1 2 2 2 2 2 (6) ( 63 ) 2 2 n1 n2 100 100
- 31. Test Concerning Means (from normally distributed data II. Two Sample Test (One Population) Test on the difference in Means B. σ2 = σ2 = σ2 are unknown 1 2 1) Ho: µ1-µ2 = µo 2) Ho: µ1-µ2 = µo 3) Ho: µ1-µ2 = µo H1 : µ1-µ2 < µo H1 : µ1-µ2 ≠ µo H1 : µ1-µ2 >µo Test Statistic: ( n 1 1 )s 1 ( n 2 1 )s 2 2 2 ( x 1 x 2 ) o where S p T n1 n2 2 1 1 Sp n1 n2
- 32. Test Concerning Means (from normally distributed data II. Two Sample Test (One Population) Test on the difference in Means B. σ2 = σ2 = σ2 are unknown 1 2 Rejection Region: T t ,( n 1 n 2 2 ) T t T t ,( n 1 n 2 2 ) ,( n 1 n 2 2 ) 2 T t ,( n 1 n 2 2 ) 2
- 33. Test Concerning Means (from normally distributed data B. σ1 = σ2 = σ2 are unknown 2 2 Example: A television network wanted to determine whether sports events or first run movies attract more viewers in the prime-time hours. It selected 28 prime-time evenings; of these, 13 had programs devoted to major sports events and the remaining 15 had first – run. The number of viewers (estimated by a television viewer rating firm) was reported for each program. If µ1 is the mean number of sports viewers per evening and µ2 is the mean number of movie viewers per evening, is there a difference in the mean number of viewers at 0.05 level of significance? The TV network’s samples produce the results below: Sports: n1 = 13 s1 = 1.8 million x 1 6 . 8 million Movies: n2 = 15 s2 = 1.6 million x 2 5 . 3 million
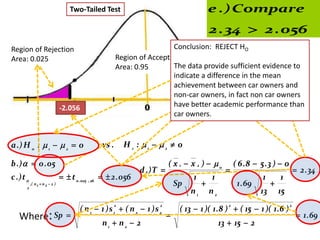
- 34. Two-Tailed Test e .) Compare 2 . 34 2 . 056 Region of Rejection Conclusion: REJECT HO Region of Acceptance Region of Rejection Area: 0.025 Area: 0.95 Area: 0.025 The data provide sufficient evidence to indicate a difference in the mean achievement between car owners and non-car owners, in fact non car owners -2.056 have better academic performance than 2.056 2.34 car owners. a .) H o : 1 2 0 vs . H 1 : 1 2 0 b .) 0 . 05 ( x 1 x 2 ) 0 ( 6 .8 5 .3 ) 0 d .) T 2 . 34 c .) t t 0 .025 , 26 2 . 056 1 1 1 1 2 ,( n 1 n 2 2 ) Sp 1 . 69 n1 n2 13 15 ( n 1 1 )s1 ( n 2 1 )s 2 ( 13 1 )( 1 . 8 ) ( 15 1 )( 1 . 6 ) 2 2 2 2 Where: Sp 1 . 69 n1 n2 2 13 15 2
Editor's Notes
- #10: tytytyt